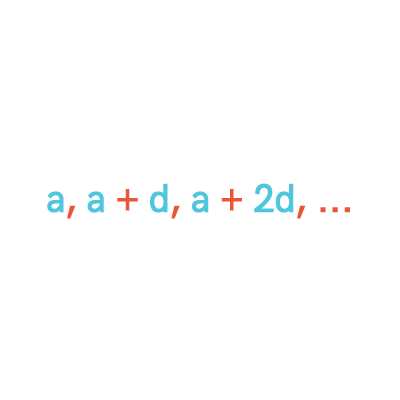Imagine you are constructing a bridge. The instructions given for the construction should be followed precisely. Lack of understanding may result in the bridge to collapse. So, measurements and accuracy play a key role in any construction. On similar lines, when it comes to construction in mathematics, it holds the same strategy. Firstly, we should …
Imagine a round cake. What is its area? By using the formula that we have studied in the lower grades, we can find the answer easily. But what if we tell you to to find the area of one slice of the cake? Is there a way to find its area? One slice of the …
Have you ever inspected an ice-cream? It reveals two solid objects, one a hemispherical scoop of delightful ice-cream, and second, the conical part that is a cone. So, this ice-cream is a combination of a cone and a hemisphere. What if we tell you to find out the volume and surface area of that entire …
Statistics is a branch of mathematics which deals with graphs, pie graphs, histograms and many more mathematical elements. In lower grades, we have already mastered skills in bar graphs, pie graphs, histograms and frequency polygons. In this chapter, we will move forward to advanced learning techniques of statistics. Here, in this lesson, you will encounter …
Ever wondered, why do meteorologists use the weather patterns to predict the chances of rain? Or how can we predict the chances of passing, in an examination, based on our performance? These ‘Chances’ that we are referring to, are nothing but ‘Probability’. In weather forecast, they check the ‘Probability of rain’, while after an examination …
The world of numbers is infinite and interesting. Till now, we have understood the concepts of natural numbers, whole numbers, integers, and rational numbers. But now the question is, are all these numbers real? That question must have surely taken your breath away, but don’t you worry. In this chapter, we take a look at …
You must be well aware of variables, such as x,y,z, and constants such as 5, ⅜, 10. Whenever you see a combination of variables and constants, there is a good chance that it might be polynomial. You will be surprised to know that polynomial is hidden everywhere in one way or another, from your phone …
In our previous grades, we have studied the methods to solve a linear equation. In this chapter, we further advance our knowledge by solving complex word problems in simpler ways. The chapter deals with finding the solution for a problem, by graphical representations as well as expressing it algebraically. Linear equations in two variables consideres …
Have you ever come across a mathematical problem which has two solutions? Woah! You must be thinking, if getting one answer was not enough, now you have to find two answers! But that’s nothing to worry, you will have so much fun learning quadratic equation. It is present in physics, for calculating the path of …
What is common between the three sequences mentioned below 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21; 6, 12, 18, 24, 30, 36, 42, 48, 54, 60, 66; -27, -24, -21, -18, -15, -12, -9. All of the above sequences are Arithmetic progressions abbreviated as AP. And what do we mean by …
© Copyright 2013 – 2020 Zouka Solutions Private Limited
